New developments in advanced welding
Weld pool behaviour
To complete a model of the GTAW process it is necessary to consider the behaviour of the liquid weld metal. The weld pool can be a very active part of the welding process, with significant energy and momentum transport taking place within it. In addition to Lorentz forces, the weld pool is subjected to variations in surface tension, buoyancy, marangoni and ‘aerodynamic’ plasma drag forces. Finally, at higher currents the pool surface can be highly distorted and this can modify current and gas flow within the arc, as well as produce another surface tension-based driver for the flow of the liquid metal (see below). In general, however, forces associated with gradients in surface tension are believed to dominate flow within the pool.
The flow resulting from gradients in surface tension is often referred to as marangoni flow (Lancaster, 1986). Normally surface tension decreases with increasing temperature, so that the weld pool surface will have a higher surface tension at the edges than at the centre. As a result the hotter weld metal at the centre is drawn across the surface to the edges, thereby establishing a circulation that transports heat directly to the edges of the pool, favouring the formation of a wide, shallow weld puddle. Under appropriate conditions this effect can be reversed by surface-active elements such as sulphur, phosphorus and selenium. These elements lower the surface tension in the cooler regions of molten metal, but are dissipated at higher temperatures. In such circumstances the temperature coefficient of surface tension can become positive (that is, surface tension could increase with temperature) and reverse the expected direction of flow. This circulation transports heat to the bottom of the pool rather than to the edges, to produce deep, narrow weld pools. In this way the performance of specific welding procedures can be compromised by heat-to-heat variations in sulphur content within a given type of stainless steel, for example. Lorentz forces also promote ‘centre-down’ circulation within the pool (see Fig. 3.3 and the discussion in Section 3.3).
When the arc current exceeds about 150 A the weld pool surface becomes noticeably concave in response to the arc forces. The degree of metal displacement increases with increasing current and becomes an important
|
(1) Electromagnetic force: fluid driven by J X B forces. |
|
(2) Natural convection (buoyancy): hot fluid under the arc spreads, cooler fluid at the edges sinks to the bottom. |
|
(3) Marangoni: surface is drawn by regions of highest surface tension (normally the coolest regions). |
|
(4) Aerodynamic drag force: surface stress arises due to friction between surface and gas stream. |
2.3 Flow directions induced by four possible motive forces in arc
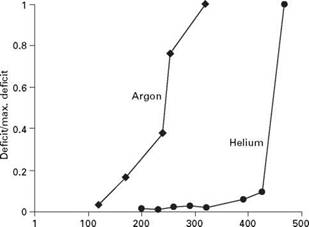
influence on process performance above about 250 A. The displacement of the weld pool is visible as a terminating crater if the weld is abruptly terminated. Such craters are interesting for several reasons. For example, their presence indicates that the liquid displaced by the arc forces does not simply accumulate around the edges of the pool but actually gets frozen into the weld bead. The amount of material required to fill the crater has been referred to as the ‘deficit’ (Jarvis, 2001). Although the shape of the crater may differ from the depression of the pool during welding, it is evident that the deficit is conserved. Hence measurement of the deficit, via the terminating crater, can be used to provide useful insights into the weld pool dynamics.
The use of measurements of deficit is illustrated by the data presented in Table 3.2 and plotted in Fig. 3.4. The data is from experiments involving GTA bead-on-plate welds on stainless steel using alternately argon and helium shielding gas. What is evident in each case is an abrupt and large increase in
|
Table 3.2 Pool displacement produced under various welding conditions on 10 mm AISI 304 stainless steel plate
|
|
Welding current (A) |
2.4 Variation in (dimensionless) deficit with current for melt-in mode GTAW.
deficit over small changes in current. These changes correspond to similarly large changes in penetration (Fig. 3.5). The implication is that an inadvertent choice of welding parameters near such transition regions could result in serious weld inconsistencies.
Models that balance arc forces against the combined effects of buoyancy and surface tension (Jarvis, 2001) could explain sudden changes in deficit.
|
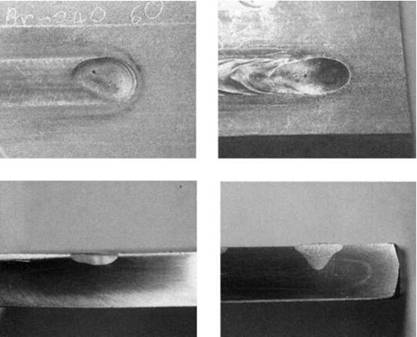
3.5 Visual evidence of abrupt changes in deficit for bead-on-plate welds on stainless steel. Both welds were made using argon shielding and at the same welding speed and voltage. Left 240 A, right 255 A. |
Essentially the argument is as follows. If the width of a weld pool is fixed and the arc force is gradually increased from zero, surface distortion will be resisted by buoyancy and by surface tension. These forces increase as the curvature increases, hence deficit rises relatively slowly. However, the resistance provided by surface tension has a maximum value (2prg) that corresponds to the surface becoming vertical at some radius r. Further increase in arc force beyond this value causes proportionately much greater displacement as it is now only limited by the weaker buoyant forces.
Models that can describe weld pool surface geometry begin with the assumption of a ‘free surface’. This means that the pool surface moves until the net pressure change across it is zero. Pressures arise from surface tension, buoyancy and arc pressure. Because the net pressure is zero everywhere the surface is at a local minimum in energy. Of course the surface is attached to the parent material at the boundary of the pool. It follows that when the boundary moves as the heat source moves along the joint, the distorted surface moves with it. (If it did not then its shape would change, its surface energy increase and it would experience a restoring force acting to realign it with the moved boundary). This automatically drives liquid metal from the leading to trailing edge of the pool and so is another potential driver for fluid flow within the pool.