EuroSun2008-5

Procedure for extrapolating performance test results

The aim was to develop a procedure with which it is possible to extrapolate the performance test results obtained by the test of only one system to other systems of the same product line. For the validation of the tool, different thermosiphon DHW systems of one product line have been tested at three different test laboratories (INETI - Instituto Nacional de Tecnologia Industrial, Lissabon, Portugal; CSTB - Centre Scientifique et Technique du Batiment, Sophia Antipolis, France; ITW, University of Stuttgart, Germany). The thermal performance data obtained by the tests of the systems and determined by the calculation tool have been compared.

2.1. Setting up a mathematical model

The mathematical model establishes a relationship between the solar fraction fsol of a solar domestic hot water system, the collector aperture area (Ac) and the store volume (Vsto),

fsol = f(A, vsto) (1)

To identify the influence of the collector size and the storage volume on the solar fraction, a product line of thermosiphon systems with the store on the roof and without auxiliary heating was modelled with the simulation software TRNSYS. The collector aperture area of the systems varies between 2 m2 and 8 m2 in steps of 1 m2 and the storage tank volumes varies between 0.2 m3 and 0.6 m3 in steps of 0.1 m3.

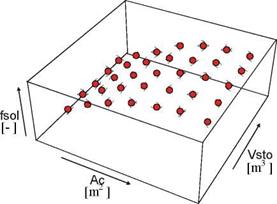
In figure 1 the values of the solar fraction are plotted depending on the collector aperture area and the storage tank volume. It can be seen that the values describe a surface in the three­dimensional space.

The surface fSol = f (Ac, Vsto ) may be described with a second order polynomial,

Fig 1. Calculated solar fraction fsol in dependency of the collector aperture area Ac and the storage tank volume Vsto

fsol(Ac, VSto) = a1 + a2 ■ Ac + a3 ■ Ac + a4 • VSto + a5 • Ac 'VS

+ a6 ■ Ac 2 ■ Fsto + a7 ■ Ac ■ VsJ + a8 ■ Vsto

where the values of a1...a8 can be determined by regression analysis. With equation (2) it is possible to calculate the solar fraction of systems with arbitrary sizes of storage tank and collector aperture area for one product line.

For each specific product line, a new function fsol = f (Ac, Vsto) has to be determined. As the

simulations necessary to derive the function are quite complex and time-consuming it is not reasonable to perform this calculation for every product line of hot water systems on the market. Hence it was necessary to find a different approach where the effects of collector aperture size and storage tank volume on the solar fraction can be calculated or at least estimated more easily.

In addition to collector aperture area and storage tank volume, there are further parameters which have an impact on the solar fraction of a thermosiphon system. The main influence parameters are:

• Geographic position (Solar radiation, mean ambient temperature)

• Performance of the thermal collector (e. g. collector efficiency)

• Performance of the storage tank (e. g. heat losses)

In order to take these different effects into account for a variety of thermosiphon systems, the main influence parameters will be classified. An example of the classification is given in Table 1.

In Figure 2, three surface plots

corresponding to equation (2) offsoi are depicted for different collector types or efficiencies, respectively. The storage volume has been kept constant in this case.

/