Application of the different test methods
A CPC collector having an aperture area of 1.87 m2 was analysed. The collector uses a circular absorber tube with an outer diameter of 19 mm. With an aperture width of 103 mm this results in a concentration ratio of C = 1.73.
Table 1 shows the results determined with the tests under quasi-dynamic and steady state conditions. The mean diffuse fraction during the test under steady state conditions was D = 0.3.
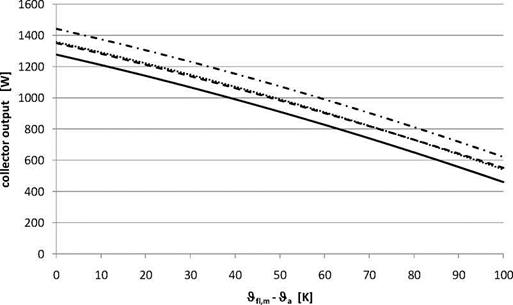
Figure 3 shows the power curves calculated using the collector parameters determined under quasidynamic conditions for diffuse fractions of 0.1, 0.3 and 0.5 together with the power curve calculated with the collector parameters determined under steady state conditions.
|
Table 1. Collector parameters determined
|
|
Figure 3 shows the significant dependency of the collector output on the diffuse fraction D. For a diffuse fraction of D = 0.5 the maximum collector output is reduced by 160 W/m2 and 11 % respectively compared to the collector output at a diffuse fraction of D = 0.1.
|
- •-D = 0.1 .......... D = 0.3 -------- D = 0.5 steady state
Fig. 3. Power curves (G = 1000 W/m2) for different diffuse fractions and under steady state conditions
The power curve determined using the test method under steady state conditions shows a similar appearance as the power curve determined for a diffuse fraction of D = 0.3. This attributes to the fact that the mean diffuse fraction during the test under steady state conditions has been D = 0.3.
From the presented investigation two main conclusions can be drawn:
1. The collector parameters gained from the test under quasi-dynamic conditions are very well suited to calculate the collector performance for different diffuse fractions. 2
The test method under quasi-dynamic conditions is, contrary to the test method under steady state conditions, very well suited to determine the thermal performance of CPC collectors having a concentration ratio larger than 1. Especially the differentiation between diffuse and beam irradiance permits a reliable modelling of the thermal performance under arbitrarily diffuse fractions. The level of detail provides a more exact estimation of the yearly energy gain and thus a better planning reliability during the dimensioning of solar thermal systems using CPC collectors.
Due to the poor reproduction of the incident irradiance the test method under steady state conditions is not suited for CPC collectors. The inaccuracy of the test method even grows with rising concentration factors.
The increasing efforts in the fields of solar thermal process heat and solar cooling have led to a rising number of concentrating collectors on the European market. Against this background it is appropriate to nominate the test method under quasi-dynamic conditions as the sole test method to be used for concentrating collectors within the next revision of the European standard EN 12975.
|
a1 |
[W/(m2K)] |
Heat loss coefficient |
|
a2 |
[W/(m2K2)] |
Temperature dependent heat loss coefficient |
|
A |
[m2] |
Aperture area |
|
C |
[-] |
Concentration ratio |
|
ceff |
[J/(m2K)] |
Effective heat capacity of the collector |
|
D |
[-] |
Diffuse fraction |
|
G |
[W/m2] |
Hemispherical irradiance |
|
Gdfu |
[W/m2] |
Diffuse irradiance |
|
Gdir |
[W/m2] |
Beam irradiance |
|
Gnet |
[W/m2] |
Useful irradiance |
|
K(0) |
[-] |
Incidence angle modifier for hemispherical irradiance |
|
Kbeam(0) |
[-] |
Incidence angle modifier for beam irradiance |
|
Kdfu |
[-] |
Incidence angle modifier for diffuse irradiance |
|
N |
[-] |
Fraction of useful irradiance |
|
Q |
[W] |
Collector output |
|
П0 |
[-] |
Conversion factor |
|
0 |
[-] |
Angle of incidence |
|
0a |
[°C] |
Ambient temperature |
|
0fl, m |
[°C] |
Mean fluid temperature |
|
t |
[s] |
Time |
[1] DIN EN 12975-2:2006, Thermal solar systems and components - Solar collectors - Part 2: Test methods -, 2006.