Char Gasification
The slowest reactions in gasification, and therefore those that govern the overall conversion rate, are the heterogeneous reactions with carbon, namely the water gas, Boudouard, and hydrogenation reactions already discussed in Chapter 2. The rates of reaction for the water gas and Boudouard reactions with char are comparable and are several orders of magnitude faster than for the hydrogenation reaction (Smoot and Smith 1985, p. 79).
There are several different models describing the Boudouard and water gas reactions (Williams etal. 2000). A widely utilized model for the Boudouard reaction is attributable to Ergun (1956) and proposes a two-step process.
Step 1 Cfas+C02 S C(O) + CO
Step 2 C(0)^C0 + Cfas
In the first step, C02 dissociates at a carbon free active site (Cfas), releasing carbon monoxide and forming an oxidized surface complex (C(O)). In the second step the carbon-oxygen complex produces a molecule of CO and a new free active site. The rate limiting step is the desorption of the carbon-oxygen surface complex.
The model for the water gas reaction is basically similar:
Step 1 Cfas+H20 5 C(O) + H2
Step 2 C(0)^C0 + Cfas
In this case the first step is the dissociation of a water molecule at a carbon-free active site (Cfas), releasing hydrogen and forming an oxidized surface complex (C(O)). In the second step, the carbon-oxygen complex produces a molecule of CO and a new free active site. In some models the rate-limiting step is the desorption of the carbon-oxygen surface complex as for the Boudouard reaction. Other models include the possibility of hydrogen inhibition by the inclusion of a third step: or
Step 3b C^ + ^H^QTf)
whereby some of the sites can become blocked by hydrogen.
Fundamental work continues in this field to develop a detailed understanding of the mechanisms of gasification reactions (Williams etal. 2000, p. 237).
Rate of Reaction. For the Boudouard reaction (2-4),
C + C02^2C0
being an equilibrium reaction, the reaction rate of carbon conversion,
= d£ r, n ~ dt
is assumed to be proportional to the concentration of C02 in the gas, so that
rm km ' С со 2
where km is the mass-related reaction-rate constant, cC02 the concentration of C02 in the gas and the order of the reaction may be assumed to be 1.
The temperature dependency of the rate constant can be expressed in Arrhenius form as
-E
km = A ■ eRT (3-3)
where A is a pre-exponential factor and E is the activation energy for the reaction. This can be expressed alternatively as
ln(km)=-f ^+ln(A) (3-4)
which provides a convenient form for comparing the reactivities of different chars.
Comparison of Different Types of Solid Feedstocks. The reactivity of different coals and chars depends on a number of factors, in particular
• The porosity of the coal, that is, its inner structure, surface, and active sites.
• The crystal structure of the fixed carbon.
• Catalytic effects of ash components in the coal.
Young (low-rank) coals such as browncoal have a large specific surface and thus a high reactivity. On the other hand, older coals, particularly anthracitic coals, have a poor reactivity. Reactivity is enhanced by alkalis, particularly potassium.
|
Figure 3-3. Reactivity of Various Materials as a Function of Temperature (Source: Biirkle 1998) |
In a systematic review Biirkle (1998) has plotted the reactivity of different chars from various biomasses, coals, and other materials, as shown in Figure 3-3.
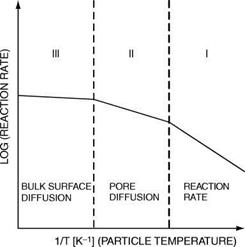
Effective Reactivities. In all cases it is necessary to distinguish between the physical and chemical steps involved and which effects control the measurable rate of reaction in different temperature zones.
Zone I, the low-temperature zone, in which the chemical reaction is the ratecontrolling step and the experimentally observed activation energy is the true activation energy.
Zone II, a medium-temperature zone, in which the rate of chemical reaction is higher, but is limited by internal diffusion of the gaseous reactants through the pores of the individual particles. The observed activation energy is only about half the true value.
Zone III, a high-temperature zone in which external, bulk surface diffusion of the gaseous reactants is rate controlling and the apparent activation energy is very small.
This is illustrated in Figure 3-4.
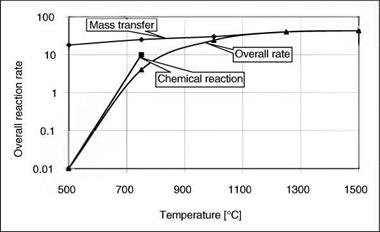
Data for coal gasification is presented in Figure 3-5, from which it can be concluded that in any solid-fuel gasification process the progress of the reaction is determined by the mass transfer phenomena.
Another indication of the importance of mass transfer is given in Figure 3-6 where the time required for the gasification of a solid fuel is plotted as a function of the particle size.
|
Figure 3-4. Effective Reaction Rates in Temperature Zones |
|
Figure 3-5. Overall Gasification Reaction Rate as a Function of Temperature (Source: Hedden 1961 with permission from Elsevier) |