Power calculations after efficiency test results
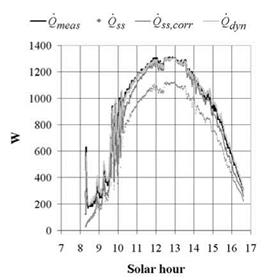
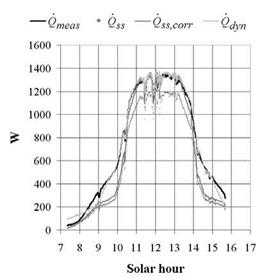
Figures 2a) and 2b) illustrate, for both measurement periods, measured (Qmeas) and calculated instantaneous power values, after both steady-state efficiency parameters (uncorrected - Qss - and corrected - Qss corr - calculations), form Eqs.(3) and (5), and dynamic (Qdyn ) efficiency test parameters, after Eq.(4).
|
a) b)
Regarding steady-state test parameters based calculations, integration of measured and calculated power curves in figure 2 yields, for the first measurement period, energy underestimations of 19.7% and 6.5% for uncorrected and corrected calculations, respectively. For the second measurement period, these results change to 21.3% and 10.8% underestimations, respectively.
More than accuracy purposes, which can not be assessed in this case considering that test parameters were produced after these same measured results, the results presented for dynamic test parameters based calculation illustrate the dynamic response of the method.
An assessment of the methodologies presented in section 2 follows directly from the comparison of instantaneous power results presented in section 5 for each of those methodologies.
The results obtained for both measurement periods reveal higher deviation from measured value, for the steady-state based calculation, whenever steep variations on irradiation conditions occur, as clearly illustrated in fig.2b) for the periods between 09.00 - 10.30 and 14.00 - 15.30. This result is in line with the base assumptions of such methodology which does not account with transient conditions, as in the dynamic methodology accounting a time dependent temperature variation term.
Considering the use of steady-state parameters, by far the most commonly available for marketed collectors, these results also clear the advantage of using the power correction methodology proposed by Horta et al. (2008). In fact, for both measurement periods, the results obtained after this methodology present closer results to measured values throughout the entire set of measurements. Lacking, in the same way, a dynamic response to steepest irradiation variations (which the power
6
correction methodology did not claimed to correct), such power correction presents particularly good results in mid-day periods, where milder variations where observed.
Test sequences of a CPC type collector were obtained allowing the application of two test methodologies, presently available for characterization of the efficiency of glazed collectors: i) steady state test methodology [EN 12975-2: section 6.1] and ii) quasi-dynamic test methodology [EN 129752: section 6.3], based on different model approaches for a solar collector and, consequently, imposing different algorithms for calculating the power (and energy) delivered by solar thermal collectors.
The different algorithms were presented, including the application of an algorithm for correction of power/energy results to steady state results as proposed by Horta et al. (2008). Application of these algorithms to two days of measured data allowed for a comparison of the results obtained with these different methodologies.
The results obtained allow the following conclusions:
• calculations based in steady-state test parameters lack dynamic response, leading to increased power underestimations under steep variation of irradiation conditions;
• calculations based in dynamic test parameters, accounting for transient conditions after adoption of a time dependent temperature variation term, reveal a closer response under such conditions;
• considering the use of steady-state parameters, by far the most commonly available for marketed collectors, the use of the power correction methodology proposed by Horta et al. (2008) leads to more accurate results, revealing better results throughout the entire set of measurements and particularly good results under irradiation conditions closer to stationarity (milder variations, as in mid-day periods).
Furthermore, and regarding the algorithm for correction of power/energy results to steady state results proposed by Horta et al. (2008), these results validate its application against measured results of independent test of a general product. The results obtained recommend its adoption in the different software tools making use of steady-state efficiency test results.
Aa collector aperture area, (m2)
aj global heat loss coefficient, (W/m2.K)
a2 temperature dependent heat loss coefficient, (W/m2.K2)
C concentration ratio
cj global heat loss coefficient, (W/m2.K)
c2 temperature dependent heat loss coefficient, (W/m2.K2)
c5 dynamic response coefficient
I beam radiation, (W/m2)
Icoi beam radiation incident on the collector aperture plane, (W/m2)
D diffuse radiation incident on the horizontal plane, (W/m2)
Dcol diffuse radiation incident on the collector aperture plane, (W/m2)
f diffuse radiation fraction
G global irradiance incident on the horizontal plane, (W/m2)
Gcoi global irradiance incident on the collector aperture plane, (W/m2)
Gcoi, ref global irradiance incident on the collector aperture plane under collector test reference
conditions, (W/m2)
K(6) beam radiation incidence angle modifier (steady-state test)
Kb(0) beam radiation incidence angle modifier (dynamic test)
Kd diffuse radiation incidence angle modifier (dynamic test)
Kaifh hemispherical diffuse radiation weighted average incidence angle modifier
q power flux, (W/m2)
Q power, (W)
q meas measured power flux, (W/m2)
Qmeas measured poweB (W)
Qdyn power calculated after dynamic efficiency curve parameters, (W)
Qss power calculated after steady-state efficiency curve parameters, (W)
Qss corr power calc. after steady-state effic. params. and power correction methodology, (W)
Rg ground reflected radiation, (W/m2)
Ta air temperature, (°С)
Tf average heat transfer fluid temperature, (°С)
в collector tilt angle, (°)
П collector instantaneous efficiency
ijo collector optical efficiency
n0b collector beam optical efficiency
в incidence angle, (°)
Єї, (в) incidence angle projection in the longitudinal (transversal) plane (°)
ez incidence angle on the horizontal plane (°)
pg ground reflectivity (albedo)
[1] Carvalho, M. J., Kovacs P., Fischer, S., Project NEGST - New Generation of Solar Thermal Systems, "WP4- D2.1.k - Resource document - Definitions and test procedure related to the incidence angle modifier”, 2006, in http://www. swt-technologie. de/WP4_D2.1_ges. pdf
[2] EN 12975-2:2006. Thermal solar systems and components - Solar collectors - Part 2: Test Methods, Section 6.1 and Section 6.3. European Standard, March 2006.
[3] Horta, P., Carvalho, M. J., Collares-Pereira, M., Carbajal, W., “Long term performance calculations based on steady state efficiency test results: analysis of optical effects affecting beam, diffuse and reflected radiation”, Solar Energy, 2008, doi:10.1016/j. solener.2008.01.004. In Press.
[4] Carvalho, M. J., Horta, P., Mendes, J., Collares-Pereira, M., Maldonado, W., 2007. “Incidence Angle Modifiers: a general approach for energy calculations”, Proceedings of ISES Solar World Congress 2007, Beijing, 18th - 21st September
[5] Mclntire, W. R., “Factored approximations for biaxial incident angle modifiers”, Solar Energy 29 (4), 315322, 1982.
[6] Rabl, A., “Active Solar Collectors and their applications”, Oxford University Press, Oxford, 1985.