Solar energy in progress and future research trends

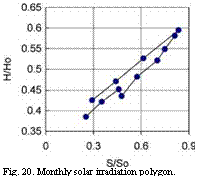
Solar irradiation polygon model

Classical approaches based on Angstrom equation for expressing the solar global irradiation in terms of sunshine durations are abundant in the literature. As already explained above, all of them include linear and to a lesser extent nonlinear relationships between these variables. The parameters in these relationships are determined invariably by the least squares technique leading to regression lines or curves as models. None of these models provides within year variations in the parameters, and they are all very rigid in the application yielding to a single solar global irradiation estimate for a given sunshine duration value. Sen and §ahin [131] have presented a solar irradiance polygon (SIP) concept for evaluating both qualitatively and quantitatively within the year variations in the solar energy variables. On the basis of monthly, seasonal and annual SIPs parameters of the classical Angstrom approach are calculated by considering nonlinear features.

Both solar irradiation and sunshine duration records depend on combined effects of astronomical and meteor­ological events. The astronomic effects on the solar energy variables are deterministically calculable by mathematical expressions depending on the average distance of the sun, longitude, latitude, declination angle at different locations and seasons of the year (Section 9). Hence, they show definite periodicities without random behaviors. Besides, solar energy related meteorological events are measured for each day as for the sunshine duration and surface global variation. The meteorological events are unpredictable and their direct effects on the solar energy calculations introduce random behaviors. For these reasons meteorological solar irradiation and sunshine duration variables have randomness in their temporal and spatial evolutions. In fact, the meteorological variability reflects itself in the astronomical extraterrestrial irradiation H0 and sunshine duration S0, i. e. length of the day in two ways.

1. The astronomical extraterrestrial irradiation, and sun­shine duration are shortened due to meteorological and atmospheric events which are measured at a solar station as meteorological solar irradiation H and sunshine duration S. In other words, S < S0 and H < H0.

2. The shortening effect is not definite but might be in the form of different and random amounts during a day or month depending on the climate and weather conditions.

Consequently, ratios of meteorological solar energy variables to astronomical counterparts as H/H0 and S/S0 assume values between 0 and 1 in a random manner depending on the cloud cover percentage of the period concerned. Furthermore, it is logically obvious that these two ratios are directly proportional to each other. In practice, the measurements of S is comparatively easier and economical than H0 and therefore, many researches have proposed various statistical expressions in order to estimate the latter from the former.

So far in the literature Angstrom linear model para­meters, a and b, are considered constant for the time period used in the application of Eq. (36). For instance, if daily values are used than a straight-line passing through the scatter of solar irradiation versus sunshine duration plots is matched which minimizes the deviation squares summation from this line, i. e. least squares technique.

Angstrom’s linear model relates the global radiation to the sunshine duration only by ignoring the other meteor­ological factors such as the relative humidity, maximum temperature, air quality, latitude, elevation above mean sea

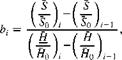level, etc. Each one of these factors contribute to the relationship between the JH/H?0 and S/S0 and their ignorance causes some errors in the model prediction and even in the linear model adaptation. For instance, the model in Eq. (36) assumes that if all the other meteorological factors are constant, then the global horizontal radiation is proportional to the sunshine duration only. The effects of other meteorological variables appear as deviations from the straight-line fit on a scatter diagram. In order to cover these error terms to a certain extent, it is necessary to assume that the model coefficients are not constant but random variables that change with time [121]. On the other hand, many researchers have considered additional meteorological factors to Eq. (36) for the purpose of increasing the accuracy in the coefficients estimate [31,47,104,107,113,120]. Although each one of these studies refined the coefficient estimates, but they all depend on the average parameter values obtained by the least squares method, and therefore, there are still remaining errors although smaller than the Angstrom’s model. On the other hand, Ogelman et al. [94] have adopted the incorporation of the standard deviation of the sunshine duration for a better estimation of the model parameters, namely, a and b. Soler [113] has shown that monthly variations of (a + b) are meteorologically sound and similar for different locations. However, he has not provided the monthly variations of a and b separately which can be obtained by SIP model.

The view taken herein, is that the linear regression technique which yields the average coefficient values is not sufficient to represent the whole variability in the meteor­ological factors, and still better interpretations within year variations should be considered from the scatter diagram. In order to achieve such a goal, the scatter of monthly average of H?/H?0 versus S/S0 ratios is considered with successive connections of months which appear in the form of irregular polygon. Hence, simple SIP concept is proposed for identifying numerically the linear variation parameters between successive months and to make useful interpret­ations from the appearances of SIPs. Since, the SIPs are closed polygons, non-linearity in within-year changes is also accounted in the solar irradiation data processing.

In search for relationship between past solar irradiance and sunshine duration data, classically a Cartesian coordi­nate system is used for the scatter of points, and then according to the appearance of these points, linear or nonlinear expressions are suggested, and subsequently, by the least squares technique the model parameters are estimated.

By considering time sequence, the points on the scatter diagram are connected successively. If this is done, say, on monthly basis, one cannot appreciate the pattern as shown in Fig. 20. No doubt, there should be a certain pattern due to at least the astronomic effects such as month to month periodic effects. A close inspection indicates that there emerges a polygon with 12 sides and vertices in a monthly sequential order which is referred to as the SIP. Features such as width,
peripheral length, side lengths, aerial extent change depending on the geomorphologic characteristic of the station site, its altitude and longitude but more significant changes depending on the weather conditions. Hence, apart from the scatter of points SIPs provide the time variations. Since, it is known physically that surface global solar irradiation is positively related to the sunshine data, all the SIPs exhibit that high (low) values of extraterrestrial solar irradiation follow high (low) values of the sunshine data. In general, these diagrams provide the following benefits over the classical models.

1. they are closed polygons which indicate that the global solar irradiation and sunshine duration processes evolve periodically within a year. However, on the top of such a periodicity, there are also the effects of the local meteorological conditions. The reason of having different SIPs at different sites is due to differences in the weather and climate conditions, in addition to longitude, latitude and altitude values;

2. each side of the polygon shows transition, i. e. variation of the solar global irradiation amount with the sunshine duration between two successive months;

3. similar to the regression straight-line concept where the slope is related to parameter b, it is possible to calculate the slope between the two successive months, say i and i — 1 as

i = 2,3,12 (59)

Herein, this coefficient is referred to as the monthly global solar irradiation change (MGSIC) with the sunshine duration. Sahin and Sen [121] have employed this equation in their study for the statistical analysis of the Angstrom parameter assessments. In fact, it is equivalent to the derivation of the global solar irradiance with respect to the sunshine duration. The smaller the time interval the closer this ratio to the mentioned derivation. It is to be noticed that such an interpretation cannot be attached to Angstrom
parameter b, the estimation of which is based on the classical regression equation;

4. another detailed information that can be deduced from SIPs is the direction in the Я/Я0 versus S/S0 variation. Since, as stated above, these polygons are close, it is, therefore, necessary that there are two possible revolution directions either clockwise or anti-clock­wise. Hence, in some monthly durations the MGSIC values become negative, in the sense that the global solar irradiation and the sunshine duration start to decrease with time. This is contrary to what can be deduced from the classical regression Angstrom coefficient b which does not provide any information about the direction of the change;

5. the lengths of polygon sides give way to weather astronomical change interpretations from one month to another. For instance, short lengths show that the changes are not significant. This is especially true if the weather conditions have remained almost the same during the transition between two successive months. For instance, one type of clear, hazy, overcast, partly cloudy and cloudy sky conditions during such a transition cause these lengths to be short;

6. comparison of two successive sides also provides information about the change of solar irradiation rate from one month to other. If the angle between the two sides is negligibly small, it is then possible to infer that the weather conditions have remained rather uniform;

7. the more the contribution of diffuse solar irradiation on the global irradiation the wider will be the SIP;

8. depending to the closeness of each side to vertical or horizontal directions, there are different interpret­ations. For instance, in the case of nearly horizontal side, there is no change in the global solar irradiance which means that the effect of weather has been such that it remained almost stable;

9. each polygon has rising and falling limbs, hence, showing two complementary periodic cycles. How­ever, the number of months in each limb might not be equal to each other, depending on the meteorological effects and the station location;

10. the SIPs provide two values for a given constant H/H0 (or S/S0) each of which lies on a different limb as referred to in the previous step. Hence, the difference between these two values yields the domain of change for the given constant value;

11. For comparison purposes one can plot two or more SIPs according to latitudes, longitudes or altitudes.